Scott Armstrong’s research webpage

I am currently working as a CNRS Directeur de recherche at Sorbonne University in the Laboratoire Jacques-Louis Lions (LJLL). I am on leave from my position as Professor of Mathematics at the Courant Institute of Mathematical Sciences at NYU.

My research focuses on mathematical physics, probability theory, and partial differential equations, with a particular emphasis on homogenization theory. This field investigates elliptic and parabolic equations, along with the associated diffusion processes, in highly heterogeneous environments.

Recently, I have concentrated on developing rigorous renormalization group methods, inspired by homogenization techniques, and applying them to problems in mathematical physics. For a high-level overview, see the short review article we wrote with T. Kuusi for Proceedings of the ICM 2026. 

Some of my recent work with T. Kuusi and A. Bou-Rabee has been featured in Quanta magazine. 

My research is supported by an ERC Advanced Grant (from Oct 1, 2025 – Sept 30, 2030). I will be considering postdoctoral candidates with strong backgrounds in probability, analysis, and mathematical physics. (A strong background in all three is not necessary.) Inquiries should be directed to scottnarmstrong+erc@gmail.com. 

Books/Monographs
  • S. Armstrong, T. Kuusi. Elliptic Homogenization from Qualitative to Quantitative. announcement | arxiv | github  
  • S. Armstrong, T. Kuusi and J.-C. Mourrat. Quantitative Stochastic Homogenization and Large-Scale Regularity. Grundlehren der mathematischen Wissenschaften vol. 352, Springer-Nature, Cham, 2019. full text
Selected Papers
  • S. Armstrong and T. Kuusi. Renormalization group and elliptic homogenization in high contrast. Invent. Math., in press. arXiv | journal | blog post
  • S. Armstrong, A. Bou-Rabee and T. Kuusi. Superdiffusive central limit theorem for a Brownian particle in a critically-correlated incompressible random drift.  arXiv | Quanta | blog post | youtube
  • S. Armstrong and V. Vicol. Anomalous diffusion by fractal homogenization. Ann. PDE, 11 (2025) art. 2, 145p. arXiv | journal | blog post | youtube
  • S. Armstrong and W. Wu. 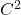C^2 regularity of the surface tension for the 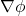\nabla\phi interface model. Comm. Pure Appl. Math., 75 (2022), 349-421. arXiv| journal 
  • S. Armstrong and P. Dario. Elliptic regularity and quantitative homogenization on percolation clusters. Comm. Pure Appl. Math., 71 (2018), 1717-1849. arXiv | journal
  • S. Armstrong, T. Kuusi and J.-C. Mourrat. The additive structure of elliptic homogenization. Invent. Math., 208 (2017), 999-1154. arXiv | journal
  • S. Armstrong and P. Cardaliaguet. Stochastic homogenization of quasilinear Hamilton-Jacobi equations and geometric motions. J. Eur. Math. Soc., 20 (2018), 797-864. arXiv | journal
  • S. N. Armstrong and C. K. Smart. Quantitative stochastic homogenization of convex integral functionals. Ann. Sci. Éc. Norm. Supér., 48 (2016), 423-481. arXiv | journal. 
    This paper received the 2017 SIAG/APDE Prize for most outstanding paper in PDE.
  • Complete list of publications