Skip to content
Preprints
- S. Armstrong, A. Bou-Rabee and T. Kuusi. Superdiffusive central limit theorem for a Brownian particle in a critically-correlated incompressible random drift. arXiv | Quanta | blog post | youtube
- S. Armstrong and W. Wu. The scaling limit of the continuous solid-on-solid model. arXiv
- S. Armstrong and R. Venkatraman. Quantitative homogenization and large-scale regularity of Poisson point clouds. arXiv
- S. Armstrong, B. Fehrman and J. Lin. Green function and invariant measure estimates for nondivergence form
elliptic homogenization. arXiv
- S. Armstrong, T. Kuusi and C. Smart. Optimal unique continuation for periodic elliptic equations on large scales. arXiv
Books/Monographs
- S. Armstrong and T. Kuusi. Elliptic Homogenization from Qualitative to Quantitative. arXiv | blog post | github
- S. Armstrong, T. Kuusi and J.-C. Mourrat. Quantitative Stochastic Homogenization and Large-Scale Regularity. Grundlehren der mathematischen Wissenschaften vol. 352, Springer-Nature, Cham, 2019. full text
Published Articles
- S. Armstrong and T. Kuusi. A coarse-graining theory for elliptic operators and homogenization in high contrast. Proc. ICM 2026, to appear. arXiv
- S. Armstrong and V. Vicol. Anomalous diffusion via iterative quantitative homogenization: an overview of the main ideas. Proc. 9th ECM, to appear. arXiv
- S. Armstrong and T. Kuusi. Renormalization group and elliptic homogenization in high contrast. Invent. Math., in press. arXiv | journal | blog post
- S. Armstrong and R. Venkatraman. Optimal convergence rates for the spectrum of the graph Laplacian on Poisson point clouds. Found. Comput. Math., in press. arXiv | journal
- S. Armstrong and V. Vicol. Anomalous diffusion by fractal homogenization. Ann. PDE, 11 (2025) art. 2, 145p. arXiv | journal | blog post | youtube
- S. Armstrong and P. Dario. Quantitative hydrodynamic limits of the Langevin dynamics for gradient interface models. Electron. J. Probab., 29 (2024) 1-93. arXiv | journal
- S. Armstrong and R. Venkatraman. Asymptotic expansion of the spectrum for periodic Schrödinger operators. SIAM J. Math. Anal., 56 (2024) 1770-1808. arXiv | journal
- S. Armstrong, T. Kuusi and C. Smart. Large-scale analyticity and unique continuation for periodic elliptic equations. Comm. Pure Appl. Math., 76 (2023), 73-113. arXiv | journal
- S. Armstrong and S. Serfaty. Thermal approximation of the equilibrium measure and obstacle problem. Ann. Fac. Sci. Toulouse Math., 31 (2022) no. 4, pp. 1085-1110. arXiv | journal
- S. Armstrong, S. Ferguson and T. Kuusi. Higher-order linearization and regularity in nonlinear homogenization. Arch. Ration. Mech. Anal., 237 (2020), 631-741. arXiv | journal
- S. Armstrong and W. Wu.
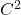 regularity of the surface tension for the
regularity of the surface tension for the 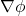 interface model. Comm. Pure Appl. Math., 75 (2022), 349-421. arXiv | journal
interface model. Comm. Pure Appl. Math., 75 (2022), 349-421. arXiv | journal
- S. Armstrong and S. Serfaty. Local laws and rigidity for Coulomb gases at any temperature. Ann. Probab., 49 (2021) 46-121. arXiv | journal
- D. Albritton, S. Armstrong, J.-C. Mourrat and M. Novack. Variational methods for the kinetic Fokker-Planck equation. Anal. & PDE, 17 (2024), 1953-2010. arXiv | journal
- S. Armstrong, S. Ferguson and T. Kuusi. Homogenization, linearization and large-scale regularity for nonlinear elliptic equations. Comm. Pure Appl. Math., 74 (2021), 286-365. arXiv | journal
- S. Armstrong, A. Hannukainen, T. Kuusi and J.-C. Mourrat. An iterative method for elliptic problems with rapidly oscillating coefficients. ESAIM Math. Model. Numer. Anal. 55 (2021), 37-55. arXiv | journal
- S. Armstrong, A. Bordas and J.-C. Mourrat. Quantitative stochastic homogenization and regularity theory of parabolic equations. Anal. & PDE, 11 (2018), 1945-2014. arXiv | journal
- S. Armstrong and P. Dario. Elliptic regularity and quantitative homogenization on percolation clusters. Comm. Pure Appl. Math., 71 (2018), 1717-1849. arXiv | journal
- S. Armstrong, T. Kuusi, J.-C. Mourrat and C. Prange. Quantitative analysis of boundary layers in periodic homogenization. Arch. Ration. Mech. Anal., 226 (2017), 695-741. arXiv | journal
- S. Armstrong and J. Lin. Optimal quantitative estimates in stochastic homogenization for elliptic equations in nondivergence form. Arch. Ration. Mech. Anal., 225 (2017), 937-991. arXiv | journal
- S. Armstrong, T. Kuusi and J.-C. Mourrat. The additive structure of elliptic homogenization. Invent. Math., 208 (2017), 999-1154. arXiv | journal
- S. Armstrong, A. Gloria and T. Kuusi. Bounded correctors in almost periodic homogenization. Arch. Ration. Mech. Anal., 222 (2016), 393-426. arXiv | journal
- S. Armstrong, T. Kuusi and J.-C. Mourrat. Mesoscopic higher regularity and subadditivity in elliptic homogenization. Comm. Math. Phys., 347 (2016), 315-361. arXiv | journal
- S. Armstrong and J.-P. Daniel. Calderón-Zygmund estimates for stochastic homogenization. J. Functional Anal., 270 (2016), 312-329. arXiv | journal
- S. Armstrong and P. Cardaliaguet. Stochastic homogenization of quasilinear Hamilton-Jacobi equations and geometric motions. J. Eur. Math. Soc., 20 (2018), 797-864. arXiv | journal
- S. N. Armstrong and J.-C. Mourrat. Lipschitz regularity for elliptic equations with random coefficients. Arch. Ration. Mech. Anal., 219 (2016), 255-348. arXiv | journal
- S. N. Armstrong, H. V. Tran and Y. Yu. Stochastic homogenization of nonconvex Hamilton-Jacobi equations in one space dimension. J. Differential Equations, 261 (2016), 2702-2737. arXiv | journal
- S. N. Armstrong and Z. Shen. Lipschitz estimates in almost-periodic homogenization. Comm. Pure Appl. Math., 69 (2016), 1882-1923. arXiv | journal
- S. N. Armstrong and C. K. Smart. Quantitative stochastic homogenization of convex integral functionals. Ann. Sci. Éc. Norm. Supér., 48 (2016), 423-481. arXiv | journal. This paper received the 2017 SIAG/APDE Prize for most outstanding paper in PDE.
- S. N. Armstrong and O. Zeitouni. Local asymptotics for controlled martingales. Ann. Appl. Probab., 26 (2016), 1467-1494. arXiv | journal
- S. N. Armstrong, H. V. Tran and Y. Yu. Stochastic homogenization of a nonconvex Hamilton-Jacobi equation. Calc. Var. Partial Differential Equations, 54 (2015), 1507-1524. arXiv | journal
- S. N. Armstrong and P. Cardaliaguet. Quantitative stochastic homogenization of viscous Hamilton-Jacobi equations, Comm. PDE, 40 (2015), 540-600. arXiv | journal
- S. N. Armstrong and H. V. Tran. Stochastic homogenization of viscous Hamilton-Jacobi equations and applications, Anal. & PDE, 7-8 (2014), 1969-2007. arXiv | journal
- S. N. Armstrong and H. V. Tran. Viscosity solutions of general viscous Hamilton-Jacobi equations, Math. Ann., 361 (2015), 647-687. arXiv | journal
- S. N. Armstrong and C. K. Smart. Quantitative stochastic homogenization of elliptic equations in nondivergence form, Arch. Ration. Mech. Anal., 214 (2014), 867-911. arXiv | journal
- S. N. Armstrong, S. Serfaty and O. Zeitouni. Remarks on a constrained optimization problem for the Ginibre ensemble, Potential Anal. 41 (2014), 945-958. arXiv | journal
- S. N. Armstrong and C. K. Smart. Stochastic homogenization of fully nonlinear uniformly elliptic equations revisited, Calc. Var. Partial Differential Equations 50 (2014), 967-980. arXiv | journal
- S. N. Armstrong and C. K. Smart. Regularity and stochastic homogenization of fully nonlinear equations without uniform ellipticity, Ann. Probab., 42 (2014), 2558-2594. arXiv | journal
- S. N. Armstrong, P. Cardaliaguet and P. E. Souganidis. Error estimates and convergence rates for the stochastic homogenization of Hamilton-Jacobi equations, J. Amer. Math. Soc., 27 (2014), 479-540. arXiv | journal
- S. N. Armstrong and P. E. Souganidis. Stochastic homogenization of level-set convex Hamilton-Jacobi equations, Int. Math. Res. Not., 2013 (2013), 3420-3449. arXiv | journal
- S. N. Armstrong and P. E. Souganidis. Concentration phenomena for neutronic multigroup diffusion in random environments, Ann. Inst. H. Poincaré Anal. Non Linéaire, 30 (2013), 419-439. arXiv | journal
- S. N. Armstrong and P. E. Souganidis. Stochastic homogenization of
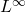 variational problems, Adv. Math., 229 (2012), no. 6, 3508-3535. arXiv | journal
variational problems, Adv. Math., 229 (2012), no. 6, 3508-3535. arXiv | journal
- S. N. Armstrong, B. Sirakov and C. K. Smart. Singular solutions of fully nonlinear elliptic equations and applications, Arch. Ration. Mech. Anal., 205 (2012), no. 2, 345-394. arXiv | journal
- S. N. Armstrong, L. Silvestre and C. K. Smart. Partial regularity of solutions of fully nonlinear uniformly elliptic equations, Comm. Pure Appl. Math., 65 (2012), no. 8, 1169-1184. arXiv | journal
- S. N. Armstrong and P. E. Souganidis. Stochastic homogenization of Hamilton-Jacobi and degenerate Bellman equations in unbounded environments, J. Math. Pures Appl. (9), 97 (2012), no. 5, 460-504. arXiv | journal
- S. N. Armstrong and L. Silvestre. Unique continuation for fully nonlinear elliptic equations, Math. Res. Lett., 18 (2011), no. 5, 921-926. arXiv | journal
- S. N. Armstrong and B. Sirakov. Nonexistence of positive supersolutions of elliptic equations via the maximum principle, Comm. PDE, 36 (2011), no. 11, 2011-2047. arXiv | journal
- S. N. Armstrong, M. G. Crandall, V. Julin, and C. K. Smart. Convexity criteria and uniqueness of absolutely minimizing functions, Arch. Ration. Mech. Anal., 200 (2011), no. 2, 405-443. arXiv | journal
- S. N. Armstrong and B. Sirakov. Sharp Liouville results for fully nonlinear equations with power-growth nonlinearities, Ann. Sc. Norm. Super. Pisa Cl. Sci. (5), 10 (2011), no. 3, 711-728. arXiv | journal
- S. N. Armstrong, B. Sirakov and C. K. Smart. Fundamental solutions of homogeneous fully nonlinear elliptic equations, Comm. Pure Appl. Math., 64 (2011), no. 6, 737-777. arXiv | journal
- S. N. Armstrong, C. K. Smart and S. J. Somersille. An infinity Laplace equation with gradient term and mixed boundary conditions, Proc. Amer. Math. Soc., 139 (2011), no. 5, 1763-1776. arXiv | journal
- S. N. Armstrong and C. K. Smart. An easy proof of Jensen’s theorem on the uniqueness of infinity harmonic functions, Calc. Var. Partial Differential Equations 37 (2010), 381-384. arXiv | journal
- S. N. Armstrong and C. K. Smart. A finite difference approach to the infinity Laplace equation and tug-of-war games, Trans. Amer. Math. Soc. 364 (2012), no. 2, 595-636. arXiv | journal
- S. N. Armstrong and M. Trokhimtchouk. Long-time asymptotics for fully nonlinear homogeneous parabolic equations, Calc. Var. Partial Differential Equations 38 (2010), 521-540. arXiv | journal
- S. N. Armstrong. The Dirichlet problem for the Bellman equation at resonance, J. Differential Equations 247 (2009), 931-955. arXiv| journal
- S. N. Armstrong. Principal eigenvalues and an anti-maximum principle for homogeneous fully nonlinear elliptic equations, J. Differential Equations 246 (2009), 2958-2987. arXiv | journal
- S. N. Armstrong and C. J. Hillar. Solvability of symmetric word equations in positive definite letters, J. Lond. Math. Soc. (2) 76 (2007), no. 3, 777-796. arxiv | journal
- S. Armstrong, K. Dykema, R. Exel and H. Li. On embeddings of full amalgamated free product
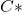 -algebras, Proc. Amer. Math. Soc. 132 (2004), no. 7, 2019-2030. arxiv | journal
-algebras, Proc. Amer. Math. Soc. 132 (2004), no. 7, 2019-2030. arxiv | journal
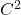 regularity of the surface tension for the
regularity of the surface tension for the 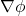 interface model. Comm. Pure Appl. Math., 75 (2022), 349-421. arXiv | journal
interface model. Comm. Pure Appl. Math., 75 (2022), 349-421. arXiv | journal 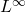 variational problems, Adv. Math., 229 (2012), no. 6, 3508-3535. arXiv | journal
variational problems, Adv. Math., 229 (2012), no. 6, 3508-3535. arXiv | journal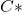 -algebras, Proc. Amer. Math. Soc. 132 (2004), no. 7, 2019-2030. arxiv | journal
-algebras, Proc. Amer. Math. Soc. 132 (2004), no. 7, 2019-2030. arxiv | journal